Cataract surgery is currently the most frequently performed surgical technique worldwide. Since the introduction of phacoemulsification by Kelman in 1967, surgical technology and construction of implanted intraocular lenses (IOLs) have undergone considerable improvement. Small, sutureless incisions and the use of foldable intraocular lenses reduced the incidence of complications and surgically induced astigmatism.1,2 Furthermore, the use of premium intraocular lenses (aspheric, toric, multifocal or a combination) allows the patient to become fully spectacle-independent.3 The improvement of surgical treatment results in rising expectations of patients. The key issue is to achieve the desired refractive outcome. Essential for this purpose are precise measurements of the eye and selection of the optimal IOL calculation formula. The aim of this article is to present current techniques of ocular biometry and IOL power calculation formulas, which will contribute to achieve highly accurate refractive outcomes.
Ocular biometry
The first step to achieve satisfactory postoperative refractive outcome is accurate ocular biometry. Biometry enables the measurement of the various dimensions of the eye, including axial length (AL), anterior chamber depth (ACD), lens thickness (LT) or central corneal thickness (CCT). These values, together with the keratometry are essential for the IOL power calculation. Precision of measurements is crucial, as a 0.1 mm error in AL results in a refractive error of about 0.27 diopter (D).4
Ultrasound biometry For many years, the only way to measure the AL of the eye was with ultrasound biometry. This technique measures the distance from the surface of the corneal apex to the internal limiting membrane (ILM). Good alignment along the ocular axis is important and that requires patient cooperation (which can be difficult in children or patients with mental disorders). In cases where a probe has direct contact with the cornea, there is a risk of a corneal damage or infection. Therefore, a topical anaesthetic and proper disinfection of the probe are required. Occurring inter-individual differences are highly dependent on the pressure exerted on the eye by the ultrasound probe. High pressure results in corneal indentation and shortening of the AL. Immersion ultrasound minimises the indentation of the cornea as it uses a saline-filled shell between the probe and the eye. Clinical studies have shown that immersion biometry is more accurate and more reliable than ultrasound biometry performed in contact mode.5–8 A limitation of ultrasound biometry is low image resolution, as a consequence of using a long, low-resolution wavelength (10 MHz) to measure small dimensions. In addition, differences in retinal thickness near the fovea or the presence of other macular pathologies contribute to inconsistent measurements.9,10
by immersion ultrasound biometry,11 but this new method is fast, easy to reproduce by different examiners, non-invasive and non-contact. Repeatability and reproducibility of measurements obtained using this technique are high and the results are less dependent on operators’ skills. However, it is difficult to obtain a measurement in the presence of a dense cataract or other opacities such as corneal scar and vitreous haemorrhage. Optical biometry measures the distance from the corneal surface to the retinal pigment epithelium (RPE). It may be associated with overestimation of measurements of about 0.15–0.5 mm.12 Optical biometry can also be successfully performed in pseudophakic or silicone oil-filled eyes. Furthermore, in high myopic eyes, due to the presence of posterior staphyloma, it may give better results than conventional ultrasound techniques for measuring the AL.
Optical biometry devices
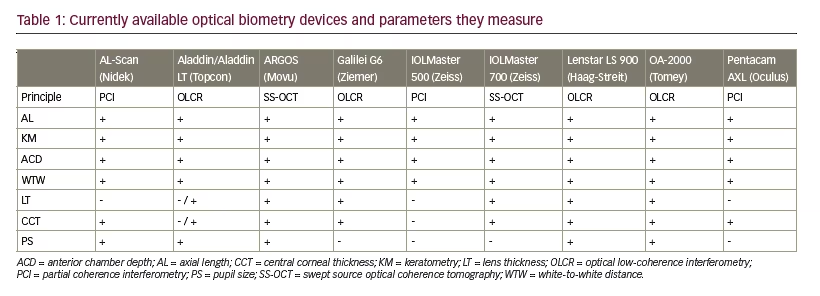
New optical biometry devices provide measurements not only of AL but also other important variables, such as: keratometry, ACD, LT, CCT, pupil size (PS) or white-to-white distance (WTW). To measure the AL of the eye, currently available devices use different technologies. IOLMaster 500 (Carl Zeiss Meditec, Jena, Germany), AL-Scan (Nidek, Aichi, Japan) and Pentacam AXL (Oculus, Menlo Park, California, US) use partial coherence interferometry (PCI) technology. Lenstar LS 900 (Haag-Streit, Koeniz, Switzerland), Aladdin (Topcon, Tokyo, Japan), Galilei G6 (Ziemer, Port, Switzerland) and OA-2000 (Tomey GmbH, Nürnberg, Germany) use optical low-coherence interferometry (OLCR). Swept source OCT (ss-OCT), used by the IOLMaster 700 (Carl Zeiss Meditec, Jena, Germany) and ARGOS (Movu, Santa Clara, California, US) devices, is the newest technology to be implemented in biometry.
The IOLMaster 500 was the first optical biometer and was introduced in autumn 1999. The device is based on the PCI principle and measures AL using infrared light (λ=780 nm) of short coherence emitted by semiconductor laser diode. Furthermore, it measures keratometry, analysing the anterior corneal curvature at six reference points at approximately 2.3 mm optical zone. The ACD is measured using slit-lamp illumination and is defined as a distance from the corneal epithelium and to the anterior lens surface. WTW is obtained by analysing the image of the iris using an infrared light source (wavelength 880 nm). All measurements are performed simultaneously. IOLMaster 500 is currently considered as a gold-standard biometer.13–15 Its repeatability and reproducibility have been assessed in several studies.16–19
AL-Scan uses an 830 nm infrared laser diode for AL measurement with PCI. It also measures keratometry (K) at 36 measurement points in two circles with diameters of 2.4 mm and 3.3 mm, reflected from the corneal surface. WTW and PS are obtained by analysing the image of the iris and fitting the best circle with the lowest error square to the detected edge. ACD and CCT are measured with an incorporated Scheimpflug camera with a 470 nm monochromatic light. The device was introduced for clinical practice in Europe in 2012. Srivannaboon et al.20 compared the repeatability and reproducibility of ocular biometry and IOL power obtained with AL-Scan and IOLMaster 500. AL-Scan provided excellent repeatability and reproducibility for all measured parameters (AL, K, ACD and WTW). Agreement with the IOLMaster 500 was good except for the WTW. This can be caused by different algorithms used by these devices for edge detection around iris image. Furthermore, the light source used for WTW measurements is different: AL-Scan uses a green light source (wavelength 525 nm) and IOLMaster uses an infrared light source (wavelength 880 nm). Kaswin et al.21 evaluated the agreement in AL, K, ACD measurements and IOL power calculations with AL-Scan and IOLMaster 500. They reported excellent correlation in AL measurements and K readings as well as good agreement in ACD measurements between these two biometers. The IOL power calculations were also highly comparable between these devices.
The Pentacam AXL device consists of a Scheimpflug camera which rotates around the eye and a PCI-based optical biometer. It was introduced in autumn 2015. In addition to anterior segment tomography, ACD, CCT and WTW measurements, corneal topography, anterior and posterior corneal surface and spherical aberrations, it also has integrated AL measurement. Calculation of toric IOLs is based on the total corneal refractive power and it takes into account the influence of the posterior corneal surface. To our knowledge, no study has yet evaluated the repeatability, reproducibility and accuracy of biometry measurements obtained using this device.
The Lenstar LS 900 biometer is based on OLCR. Using a 820 μm superluminescent diode as light source, it allows the measurement of the AL, CCT, LT and ACD. The retinal thickness can also be determined from the scans, but this requires subjective alignment of a cursor. It also uses 950 μm light to assess by image analysis central corneal curvature using two rings of diameter 1.65 mm and 2.30 mm of 16 light spot each. WTW and PS are obtained by fitting the best circle with the lowest error square to the detected edge. Optional T-cone module complements this device with a Placido topography of the central 6 mm corneal zone. Several studies confirmed Lenstar’s repeatability, reproducibility and agreement with other biometry devices. Generally, Lenstar provided results that correlated very well with those of the IOLMaster. Excellent agreement has been shown between the AL measurements taken by Lenstar and IOLMaster,22–25 but only good22 or moderate24 agreement
Optical biometry The introduction of optical biometry has steadily replaced ultrasound methods and is now considered the clinical standard for ocular biometry. The results are comparable to those achieved between these two devices in ACD measurements. In some cases, small but statistically significant differences in K and ACD measurements were reported.24 However, in a few studies, the AL measurements taken by Lenstar were slightly higher than the IOLMaster measurements, but the differences were not clinically significant.22,23 The Lenstar was unable to take measurements due to lens opacities in a similar number of patients to the IOLMaster.23
Aladdin is an optical biometer based on OLCR with an 830 nm super luminescent diode and Placido topography system. It allows to perform eight measurements in one acquisition: AL, keratometry, corneal topography, ACD, pupillometry, WTW, CCT and LT, although the last two parameters (measured by OLCR) are available only on the Aladdin LT. Pupillometry can be measured in three modes: dynamic, photopic and mesopic. Corneal topography is based on the reflection of 24 Placido disc rings with a diameter of 8.0 mm. Topography-based keratometry is obtained by analysing approximately 1,024 data points of four dedicated Placido rings whose diameters range between 2.4 mm and 3.4 mm. Aladdin provides also Zernike analysis and keratoconus screening. In several studies, Aladdin provided good agreement and repeatability compared with the IOLMaster. According to Huang et al.,28 repeatability and reproducibility for AL, ACD and K measurements was found to be excellent. However, the precision of WTW measurements was lower in eyes with cataract. In addition, Aladdin is equipped with Placido-disc corneal topographer and can provide information that is not available on the IOLMaster, such as corneal map and corneal asphericity, which were recently shown to influence the IOL power refractive prediction error.29
OA-2000 combines Placido-disc topography and OLCR biometry. It measures AL, CCT, LT and ACD using the OLCR technique. Corneal curvature is measured by Placido-disc topography with nine rings each 256 points in a 5.5 mm zone projected onto the cornea. It also measures WTW and PS. Goebels et al.30 compared the OA-2000 device with the Lenstar and IOLMaster. In this study, the OA-2000 biometer generated the most accurate results that correlated very well with the measurements obtained by Lenstar and IOLMaster. Excellent correlation among all three devices was shown for AL measurements. Although three different techniques to achieve K values were used, the correlation between the different devices was very high. For ACD measurements, good correlation was found, with the highest correlation between OA- 2000 and Lenstar devices (both use OLCR). The ACD values were highest with the OA-2000 and lowest with the IOLMaster. All differences were statistically, but not clinically, significant.
Galilei G6 combines OLCR optical biometry, dual-Scheimpflug imaging and Placido-disc topography measures AL, LT, ACD, CCT, corneal topography, PS and WTW. In addition to biometry, Galilei G6 provides high-definition pachymetry, total corneal wavefront, curvature and astigmatism data of the anterior and posterior cornea – complete data required to plan cataract or refractive surgery. Shin et al.31 compared Galilei G6 with Lenstar biometer. All parameters measured by the Galilei G6 were highly repeatable. There were no statistically significant differences between K and ACD measurements obtained by these two devices, however, the measurements for AL, LT and WTW were significantly different. The K, AL, ACD, LT and WTW showed good correlations (all p<0.001), however, the agreements of LT and WTW were not good between the two devices. The IOL powers using the SRK/T, Holladay 1, Hoffer Q and Haigis formulas were compared – they did not show statistically significant differences (all p>0.05), however, agreements between the IOL powers were not strong. IOLMaster 700 is a biometry device based on swept-source OCT technology that enables full-eye length tomography, providing good fixation control. It uses high-frequency 1,055 nm tunable laser source for AL, LT, ACD and CCT measurements. Keratometry measurements are distance-independent. Light is projected onto the cornea at three zones (1.5, 2.5 and 3.5 mm). PS and WTW are obtained using an LED light source. Furthermore, the device provides a 1.0 mm horizontal scan of the retina to ensure that the measurements are on the visual axis by using the presence of foveal pit.32 According to Srivannaboon et al.,32 the measurement speed of IOLMaster 700 was statistically significantly faster than IOLMaster 500 (p<0.05). In several studies, IOLMaster 700 showed very high repeatability and reproducibility and good agreement with IOLMaster 500 and Lenstar,32–34 although repeatability and reproducibility of ACD measurements obtained by IOLMaster 700 were better than those from the IOLMaster 500.32 In addition, studies showed that IOLMaster 700 penetrated the opaque media better and measured the AL with fewer dropouts compared with the Lenstar and IOLMaster 500 even in dense cataracts.32,34
Argos biometer uses a 1,060 nm wavelength and 20 nm bandwidth swept-source technology to collect two-dimensional OCT data of the full eye (SS-OCT). It measures AL, LT, ACD and CCT with ss-OCT. Keratometry values are generated by illumination from a ring of 16 infrared LEDs. In addition, the device measures PS by analysing the two-dimensional OCT image. Shammas et al.35 evaluated the repeatability and reproducibility of the measurements obtained with the Argos biometer and compared them with the results obtained with the IOLMaster 500 and the Lenstar LS 900 biometers. The study showed high repeatability and reproducibility of measurements obtained by Argos biometer. AL measurements with the new SS-OCT biometer were comparable to PCI and OLCR measurements, with a faster and higher acquisition rate, even in the presence of a dense nuclear or posterior subcapsular cataract.
Optical measurements differ from measurements obtained by ultrasound methods. Therefore, individual optimisation of constants is necessary. This can be achieved by thorough analysis of pre- and postoperative clinical data. In October 1999, an independent group of scientists and users, working in the field of optical biometry founded the User Group for Laser Interference Biometry (ULIB). One of the most important purposes of this group is the optimisation of lens constants for the IOL power calculation. The results of this optimisation are published on the ULIB website (http://ocusoft.de/ulib/index.htm). Optimised IOL constants are currently available for the IOLMaster 500 and 700, Lenstar LS 900, AL-Scan, Aladdin and recently also for Pentacam AXL.
Intraocular lens power calculation formulas
The original first-generation formulas are either theoretical, based on an optical model of the eye and mathematical principles, such as the Binkhorst formula, published in 1975,36 or regression formulas, based on analysis of postoperative patient refractions, such as the SRK formula developed by Sanders, Retzlaff and Kraff in 1980.37
Binkhorst’s formula: D=1336 (4r-a)/(a-d) (4r-d)
D = power of IOL in aqueous humor; 1336 = index of refraction of vitreous and aqueous; r = radius of curvature of the anterior surface of the cornea; a = axial length of the eye; d = distance between surface of the cornea and the IOL
SRK formula: P=A-2.5L-0.9K P = power of lens for emmetropia; A = A-constant of the IOL; L = axial length; K = average keratometry
Refractive outcomes after the use of first-generation formulas were quite accurate for patients with average eye length, but often led to refractive errors in the case of very short or very long eyes.38 The SRK formula tends to calculate stronger than optimal IOL power for long eyes and weaker IOL power for short eyes.
Further analysis of clinical data was necessary. It enabled the development of more precise SRK II formula (second-generation formula) in 1988.39 This formula include a modified A constant, related to the length of the eye:
if AL<20 mm A1 = A + 3
21 mm>AL≥20 mm A1 = A + 2
22 mm>AL ≥ 21 mm A1 = A + 1
24.5 mm>AL≥22 mm A1 = A
24.5 mm>AL A1 = A – 0.5
Despite this improvement, first- and second-generation formulas are now considered to be obsolete and should be avoided in clinical practice. Third- and fourth-generation formulas should be chosen instead.
Third-generation formulas, including Hoffer Q, Holladay1 and the SRK/T, are a merger of the regression and theoretical formulas. They all use a thin-lens model that treats IOLs as thin lenses with only one effective lens plane. These formulas rely on two variables (AL and K) and can be optimised by adjusting by a suitable factor – the ‘surgeon factor’ – for the Holladay1 formula, the ACD-constant for the Hoffer Q formula and the A-constant for SRK/T formula. Terzi et al.,40 Haigis41 as well as Petermeier et al.,42 have proven that the optimisation of lens constants has made the IOL power calculation more accurate and has decreased the postoperative refractive error. The Hoffer Q formula developed in 1992 depends on personalised ACD, AL and K in order to calculate the postoperative effective lens power.43 The Holladay1 formula, published in 1988, requires a surgeon factor, which is the distance between the iris and the IOL, where the distance between the cornea and iris plane is calculated as the height of the corneal curve.44
The SRK/T formula introduced in 1990 requires an estimated ACD as a function of corneal curvature and AL with A-constant.45 A constant is a theoretical value, which connects IOL power to AL and keratometry and depends on many factors such as manufacturer, style and location of the IOL. It is specific to the design of the IOL and its intended orientation in the eye.
Fourth-generation formulas have promulgated since the 1990s and include the Haigis, Holladay2, Olsen and Barrett Universal II. These require more variables for IOL power calculation. One thing they have in common is the need to predict the non-measurable, virtual factor – effective lens position (ELP), not the anatomical one.
The Haigis formula uses three constants: a0, a1 and a2 to calculate ELP, where:
d = a0 + (a1 × ACD) + (a2 × AL)
AL and anterior depth chamber, which are measured, rather than estimated, are required, in contrast to other formulas. The Haigis formula for intraocular lens calculation requires corneal radii of curvature in millimeters instead of keratometry in diopters.46
In Olsen’s formula, the ELP is predicted primarily through a concept called the C constant, where accurate, ray tracing-assisted measurements of LT and ACD are combined in order to calculate ELP in the most effective way.47 The Holladay2 formula was proposed in 1993, when a new theory about biometry using AL and anterior segment appeared.48 This formula needs seven variables to calculate effective lens positon: AL, keratometry, WTW, preoperative refraction, ACD, LT and patient age, in order of importance.49 Barrett Universal II is a thick lens formula, in which ELP is characterised by LF (lens factor) and anatomic chamber depth. The LF is influenced by: keratometry, AL, ACD, LT and WTW, in order of importance. This formula notifies the change in planes, which is connected with different IOL powers. It recognises the negative value of lens factor in the presence of negative-powered type of IOL, which has to be taken into consideration when calculating the ELP.50
A major challenge is IOL power calculation in patients who have undergone refractive surgery, as it is difficult to measure the true corneal power and estimate the ELP. After myopic refractive surgery (photorefractive keratectomy [PRK], LASIK, radial keratotomy [RK]), both keratometry and corneal topography tend to overestimate corneal power. This problem can be remedied by double-K modifications of third-generation formulas: SRK/T, Hoffer Q or Holladay1. Double-K methods use the preoperative K values for the ELP calculation and the postoperative K values for the IOL power determination.51 One of the drawbacks of these methods is ELP calculation dependence on the central corneal power.
Other formula used for IOL power prediction after LASIK or PRK is the Masket method. This formula omits the double K step required by other pre-LASIK/PRK K-dependent methods and simply adjusts the power of the IOL using the knowledge of the surgically induced refractive change. It is particularly useful when corneal power before refractive surgery is unavailable, but the refractive change is known (even if uncertain).52 More reliable methods of determining IOL power after refractive surgery do not rely on historical data, which may be inaccurate or unavailable, for example, Shammas no-history, Haigis-L and Camellin-Calossi.
The Shammas no-history formula was first published in 2007. It is a post-LASIK modification of a previously described formula, in which the average corneal power, K, is replaced by the corrected mean corneal power, Kc and where Kc=1.14 Kpost-6.8, with Kpost being the post-LASIK K-readings in diopters.53 The Haigis-L formula, designed in 2008, using corneal radius measured in mm generates a corrected corneal radius, which is then used by the regular Haigis formula to calculate the IOL power.54
The Camellin-Calossi formula, first published in 2006, is one of the most recent formulas used commonly for calculating IOL power in eyes which have undergone refractive surgery. This formula is based on modified Binkhorst II formula and empirically adjusts corneal power and calculates ELP regardless of corneal keratometry (K). According to Suto et al., the Camellin-Calossi formula can be also used for calculating IOL in normal cataractous eyes and its accuracy is equivalent to common IOL formulas: SRK/T and Haigis.55
There are also formulas designed exclusively for particular devices, for example, PotvinShammasHill and PotvinHill formulas. They use data from the Pentacam device, specifically the true net power in a 4.0 mm zone centered on the corneal apex, to calculate IOL power in post-myopic LASIK eyes (PotvinShammasHill formula) and after radial keratotomy (PotvinHill formula).56
Recently, a new software using numerical ray tracing for IOL power calculation became available (e.g., Okulix, EasyIOL, PhcoOptics). The accuracy of numerical ray tracing is independent of AL. Therefore, very long or very short eyes can gain the most from the higher accuracy of this approach. For average-size eyes, however, the results of ray-tracing methods were as accurate as theoretical thin-lens formulas.57,58
One of the most recent calculation methods was released in June 2016: the Hill-RBF on-line calculator. It is an advanced, self-validating method using artificial intelligence and pattern recognition to select an IOL for a patient. The calculator is entirely data-driven and is independent of the limitations of theoretical vergence formulas. It has been optimised for use with the Lenstar LS 900, but may also be used with data from other optical biometers.59
It has not been proven that any of the recent formulas are better than the others, however, first and second generation formulas such as SRK II should not be used any longer, as they have minimal theoretical value.47 The new formulas are more precise than previous ones, but their advantage can be noticed clearly in IOL calculation of nontypical eyes.60 Modern IOL power calculations have similar outcomes in eyes with average AL but they are less accurate in eyes with long or short AL.43
Apparently, there is no multipurpose formula for every type of eye, the use of a particular formula depends on several parameters, such as the eye’s AL, astigmatism, previous refractive surgery and differs in phakic and pseudophakic eyes.51 According to Wang et al., the Haigis, Hoffer Q, Holladay1 and SRK/T formulas are equally accurate for calculating the IOL power in phakic eyes between 22 mm and 24.5 mm length.61
In a group of patients with AL below 22 mm, getting a precise postoperative refraction is more difficult than amongst other patients, as short eyes usually need a high-power intraocular lens. In 2012, Day et al.62 showed that the Hoffer Q formula had the lowest absolute mean error in eyes with AL from 20.00 to 20.99 mm and Hoffer Q and Holladay1 formulas had made more accurate calculations than SRK/T formula. Carifi et al.63 compared the refractive results among various formulas (Hoffer Q, Holladay1, Holladay2, Haigis, SRK-T and SRK-II) in patients undergoing phacoemulsification cataract surgery with a single highly powerful IOL implanted in the capsular bag (range of powers +35.0 to +40.0 D). The study showed that none of the latest-generation formulas (Hofer Q, Haigis, Holladay1 and Holladay2) significantly outperformed the others (p=0.245). However, the SRK formulas yielded less accurate predictions in these cases. The authors suggested that the SRK/T formula should not been used in IOL power calculation in eyes with AL shorter than 22 mm. Currently, the most recommended formulas for IOL power calculation for short eyes are the third-generation formula Hoffer Q64,65,43 and the fourthgeneration formula Holladay2.66 According to the study conducted in 2014 by Eom et al.,43 the Hoffer Q and Haigis formulas are similarly accurate in calculating IOL power in eyes with short AL, but the Haigis formula is more precise in eyes with ACD <2.4 mm. Similar outcomes were presented by Maclaren et al.67 in 2007, when they showed that the Haigis and Hoffer Q formulae performed well in eyes with long AL when using conventional biometry methods and phacoemulsification.
There are also difficulties in choosing the most appropriate IOL power for patients with high myopia. The main problem is staphyloma, which makes the measurement of AL harder than usual, as well as restricted access to IOL power calculation formulas for those patients. It was suggested that there are no significant differences in IOL power calculation in patients with AL >26 mm using Haigis, HofferQ, SRK/T formulas, but it has been shown that SRK/T formula has the lowest mean error.68 Aristodemou et al.69 has also shown that the most suitable formula for eyes longer than 27 mm is SRK/T. In 2015 it was shown that Barrett Universal II, one of the most recent published formulas, is more accurate than other known formulas in long eyes with AL greater than 26 mm.70
Patients’ requirements concerning visual effect after cataract surgery are rising. In order to increase the accuracy of IOL power calculation and postoperative refractive outcome, an ideal calculation formula has been searched for for many years. A multipurpose formula, which can be used in every eye’s AL, is still to be found. Conclusion The latest biometry technologies and modern IOL power calculation formulas have significantly improved refractive outcomes after cataract surgery. Well-calibrated devices, using optical rather than ultrasound biometry, optimised IOL constants and properly selected last-generation IOL power calculation formulas that fit to a particular patient can provide excellent refractive outcomes.







